Polarization upon Specular Reflection

正如前一节结束的时候提到的,specular reflection can change the polarization state of light. Consider two optically homogeneous, isotropic media separated by an optically smooth planar interface. Strictly speaking both media should be infinite for theory to be applicable, but this ideal (and unobtainable) condition is satisfied to good approximation if the dimensions of the media are much larger than the wavelength of the light of interest. A plane wave with wave vector k; = —k(sin -¡ey + cos-; ez) (7.87)

is incident on the interface from a negligibly absorbing medium (e.g., air) with wavenumber k. This wave gives rise to (i.e., excites) a reflected wave with wave vector kr and a transmitted wave with wave vector kt:

kr = —k(sin-rey — cos-rez), kt = —kt(sin-tey + cos-tez), (7.88)

where kt is the wavenumber in the transmitting medium. The plane of incidence, which we take to be the yz-plane (Fig. 7.5), is determined by the normal to the interface and the incident

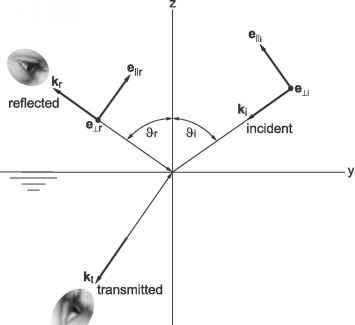
Figure 7.5: A plane wave illuminating the optically smooth interface between optical homogeneous (infinite) media gives rise to (specularly) reflected and transmitted (refracted) waves.

wave vector. All three wave vectors lie in the plane of incidence. Moreover, the angle of reflection equals the angle of incidence (law of specular reflection)

= à, and êt is given by the law of refraction (Snel's law)

where m is therefractive indexof the transmitting medium relative to that of the incident medium. Because m may be complex, so may be the angle of refraction, but the real part of this complex angle is not the angle of refraction defined as the angle between the real part of the complex wave vector and the normal to the interface. When m is complex, the transmitted wave is inhomogeneous (except fornormal incidence, = 0): the real and imaginary parts of the complex wave vector are not parallel.

Despite what you see elsewhere, the spelling of Snel here is correct. Whatever fame Snel may deserve for discovering (empirically) the law of refraction, which has been disputed, he likely has the dubious honor of having his name misspelled more than that of any other scientist in history. Kirchhoff probably runs a close second. A resurrected Snel, upon seeing his name in hundreds of textbooks and thousands of papers, might exclaim, "What the 1!", whereas Kirchhoff's reaction might be, "Where the h?"

The incident and reflected electric field components are specified relative to orthogonal basis vectors parallel and perpendicular to the plane of incidence (Fig. 7.5), defined such that x e|| is in the direction of the wave vector. Note that the parallel basis vector for the incident field is not the same as that for the reflected field. The complex field components of the reflected field relative to those of the incident field are

Ei|r m cos — cos tan($i — $t)

11 E||i m cos + cos$t tan($i + $t)

r= - = - = --(l.yL)

E±i cos + m cos $t sin(^i + $t)'

where subscripts i and r denote incident and reflected, respectively. Equations (7.91) and (7.92) are the Fresnel coefficients. Derived before the electromagnetic theory of light had been developed (Fresnel died in 1827), they specify the amplitude and phase of the reflected field for any angle of incidence and illuminated medium. The corresponding reflectivities for the two orthogonal polarization states are

R = |r|||2 , = |f±|2 . (7.93)

Underlying Eqs. (7.91) and (7.92) is the additional assumption that both media are nonmagnetic at the wavelength of interest. At normal incidence (^ = 0°) both reflectivities are equal,

and at glancing incidence (^ = 90°) both are 1 for arbitrary m. But for all intermediate angles of incidence the two are different, as, for example, those for an air-water interface illuminated by visible light (Fig. 7.6). In particular, R| =0 if n whereas R± does not vanish for any angle of incidence. From Eq. (7.90) it follows that Eq. (7.95) is equivalent to tan ^ = m. (7.96)

Equation (7.96) can be satisfied strictly only for m real.

Because R| = 0 for the angle of incidence satisfied by Eq. (7.96), unpolarized light reflected at this angle is 100% linearly polarized perpendicular to the plane of incidence. This angle is called the polarizing angle or the Brewster angle to honor Sir David Brewster, who first discovered it empirically. Brewster's paper, published in 1815, has aged well. Written with a clarity almost banned from scientific writing today, it ends with a touching homage to Etienne-Louis Malus, who discovered polarization upon reflection in 1809, coined the term

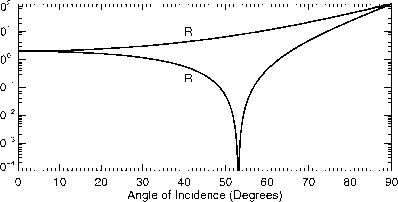
Figure 7.6: Reflectivities of water at visible wavelengths (n = 1.33) for incident light polarized parallel (||) and perpendicular (±) to the plane of incidence.

极化的(错误的)类比与杂志netic poles, but failed to recognize the regular law later enunciated by Brewster: "The index of refraction is the tangent of the angle of polarisation." Brewster measured the polarizing angle for more than a dozen transparent substances and compared its value calculated from the tangent equation, finding agreement, on average, to within 15'. But Brewster knew that he hadn't derived this equation: "In these enquiries I have made use of no hypothetical assumptions...the language of theory has been occasionally employed, but the terms thus introduced are merely expressive of experimental results. ..When discoveries shall have accumulated a greater number of facts, and connected them together with general laws, we may then safely begin. ..to speculate respecting the cause of those wonderful phenomena which light exhibits under all its various modifications." These "general laws" had to wait a few more years for Fresnel and half a century for the glorious synthesis by Maxwell.

In a paper with the provocative title "Would Brewster recognize today's Brewster angle?", Akhlesh Lakhtakia critically examined the ways in which the term Brewster angle is used today. In modern textbooks it is defined most often by the condition R\\ = 0, but Brewster would not have known this and to him the angle now bearing his name is the angle of incidence (polarizing angle) for which reflected light is completely linearly polarized given incident unpolarized light. Moreover, Lakhtakia showed that there are isotropic media for which the polarizing angle and the angle of zero reflection for the parallel component are not the same.

You sometimes encounter the assertion that reflection by metals does not polarize incident unpolarized light. Although Eq. (7.96) does not have a real solution when the imaginary part of m is not negligible, metals do exhibit an angle of reflection, sometimes called the pseudo-Brewster angle, at which the degree of polarization is a maximum. And it can be surprisingly high, well over 50%, especially for metals (e.g., iron and chromium) with reflectivities lower than those of more conductive metals such as silver and aluminum. One reason for the misconception that light reflected by metals is unpolarized is that the pseudo-Brewster angle is typically within 10° or so from glancing incidence.

Yet another misconception about polarization is that light emitted by incandescent bodies is unpolarized, which may have its origins in the fact that radiation emitted by blackbodies (which don't exist) is unpolarized. Consider an optically smooth and homogeneous medium in air, sufficiently thick that transmission by it is negligible. With this assumption, emissivity is 1 minus reflectivity (see Sec. 1.4.1), and because reflectivity depends on polarization so does emissivity:

£|l = 1 — R\\, eL = 1 — Rl. (7.97)

These twoemissivitiesare equal for normal and glancing directions, but for real bodies are unequal in all intermediate directions. Both are 1 in all directions for a blackbody (R| = Rl = 0).

Although the emphasis in this section is on polarization upon specular reflection, because it is relatively easy to observe, the state of polarization ofincident radiationalso can be changed upon transmission. The Fresnel coefficients for reflection, Eqs. (7.91) and (7.92), are accompanied by two for transmission:

E^i cos -; + m cos -t where the subscript t denotes transmission.

Continue reading here:Polarization by Dipolar Scattering Skylight

Was this article helpful?

0 0